Browse Articles

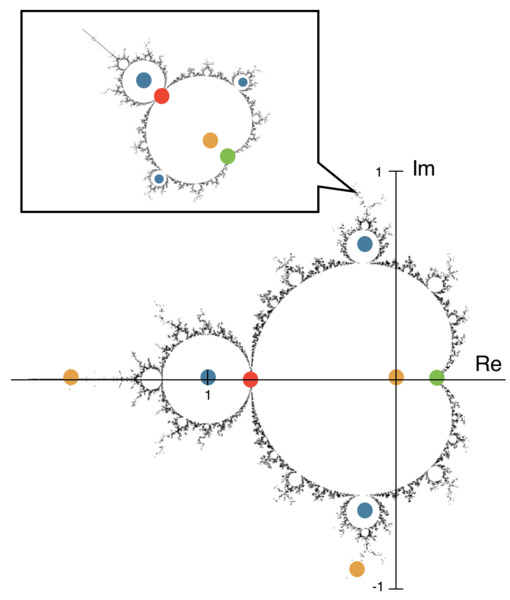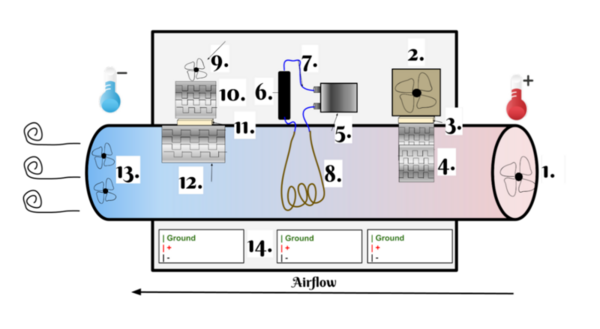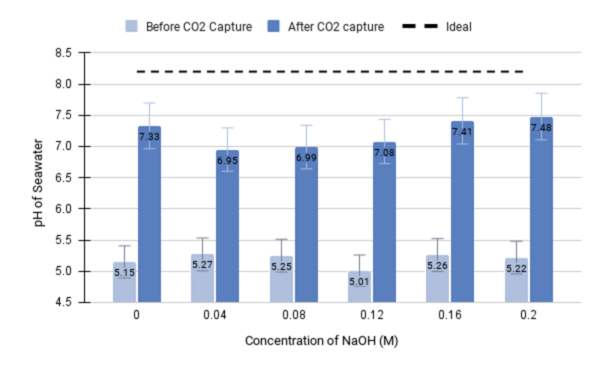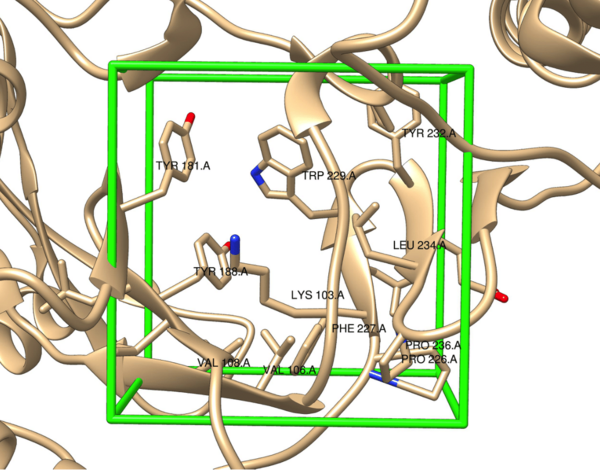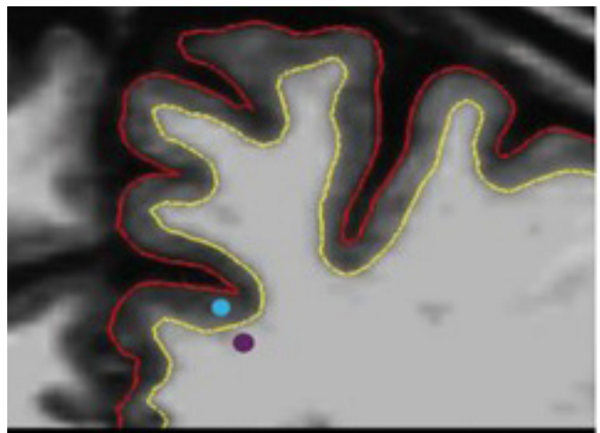
This study uses neuroimaging to investigate cognitive set-shifting, a type of executive function that involves shifting from one task to another. This study tested whether cortical gray-white matter contrast in subregions of the prefrontal cortex (PFC) was associated with set-shifting abilities in adults.

This study aimed to predict and explain chaotic behavior in the Mandelbrot Set, one of the world’s most popular models of fractals and exhibitors of Chaos Theory. The authors hypothesized that repeatedly iterating the Mandelbrot Set’s characteristic function would give rise to a more intricate layout of the fractal and elliptical models that predict and highlight “hotspots” of chaos through their overlaps. The positive and negative results from this study may provide a new perspective on fractals and their chaotic nature, helping to solve problems involving chaotic phenomena.

In this study, the authors analyze gene expression datasets to determine if there is a core set of genes dysregulated during nonalcoholic steatohepatitis.

Here based on an interest in fractals, the authors used a Julia Set Generator to consider a specific point on the Mandelbrot set with an associated coordinate. In this manner, they found that the complexity of the Mandelbrot and Julia Sets are governed by relatively simple rules, revealing that the intricate patterns of fractals can be defined by defined by simple rules and patterns.

The authors set to test a system that would help with the dehumidification and overall management of greehouses.

The authors set out to develop an electrochemical device that would have efficient and sustained carbon dioxide capture.

In this study, the authors use high-throughput virtual screening to design and evaluate a set of non-nucleoside reverse transcriptase inhibitors for binding affinity to the protein reverse transcriptase. These studies have important applications toward HIV therapies.

The authors investigate the ability of machine learning models to developing new drug-like molecules by learning desired chemical properties versus simply generating molecules that similar to those in the training set.

Supernovas are powerful explosions that result from gravitational collapse of a massive star. Using photometric analysis Arora et al. set out to investigate whether 2020pni (located in galaxy UGC 9684) was a supernova. They were ultimately able to identify 2020pni as a Type II-L supernova and determine it's distance from earth.

The facial integument, or external skin tissues, were assessed on set of dinosaurs from the Allosauroidea clade to test whether dermal patterns served specific functions.