Discovery of the Heart in Mathematics: Modeling the Chaotic Behaviors of Quantized Periods in the Mandelbrot Set

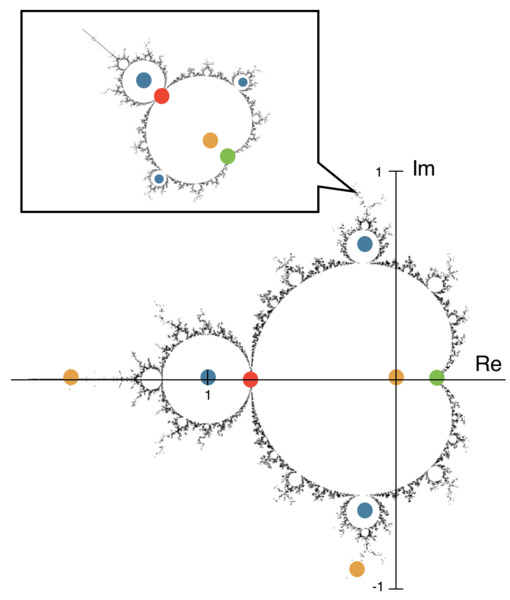
The phenomenon of small changes leading to drastic effects is fundamental to Chaos Theory. Understanding the chaos in our world could provide more control over the systems that govern the universe. Therefore, this study aimed to predict and explain chaotic behavior in the Mandelbrot Set, one of the world’s most popular models of fractals and exhibitors of Chaos Theory. We hypothesized that repeatedly iterating the Mandelbrot Set’s characteristic function would give rise to a more intricate layout of the fractal and elliptical models that predict and highlight “hotspots” of chaos through their overlaps. While a novel method of discovering miniature versions of the Mandelbrot fractal was discovered and a statistically significant transformation function was developed, overlaps of the elliptical models were not supported to exhibit higher levels of chaos. Many biological and natural phenomena such as the heartbeat, lung vessels, neurons, weather, the stock market, and more, are both chaotic in nature and can be described using fractal-based models. The positive and negative results from this study may provide a new perspective on fractals and their chaotic nature, helping to solve problems involving chaotic phenomena.