Browse Articles

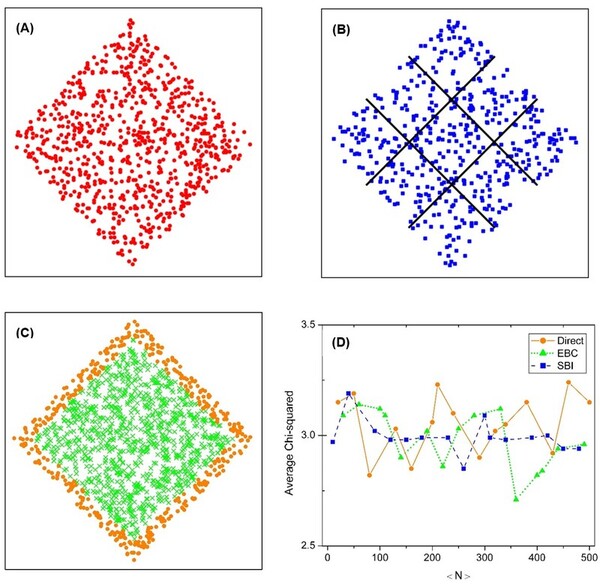
The causal set theory (CST) is a theory of the small-scale structure of spacetime, which provides a discrete approach to describing quantum gravity. Studying the properties of causal sets requires methods for constructing appropriate causal sets. The most commonly used approach is to perform a random sprinkling. However, there are different methods for sprinkling, and it is not clear how each commonly used method affects the results. We hypothesized that the methods would be statistically equivalent, but that some noticeable differences might occur, such as a more uniform distribution for the sub-interval sprinkling method compared to the direct sprinkling and edge bias compensation methods. We aimed to assess this hypothesis by analyzing the results of three different methods of sprinkling. For our analysis, we calculated distributions of the longest path length, interval size, and paths of various lengths for each sprinkling method. We found that the methods were statistically similar. However, one of the methods, sub-interval sprinkling, showed some slight advantages over the other two. These findings can serve as a point of reference for active researchers in the field of causal set theory, and is applicable to other research fields working with similar graphs.

Someday, rockets from Earth may be launched towards worlds beyond our solar system. But will these rockets be able to reach their destination within a human lifetime? Ramaswamy and Giovinazzi simulate rocket launches to an Earth-like exoplanet to uncover whether it's physically possible to complete the journey within a lifetime.

Here the authors investigated the optical possibilities of gelatin and acrylic in regards to potential implementations at soft contact lenses. They fabricated lenses of different shapes and evaluated the refraction of laser light finding that gelatin needed to be thickened or increased in curvature to account for its lower refractive index compared to plastics, or used in a mixture to strengthen the lens.

The energy conservation in a system of objects in collision depends on the elasticity of the objects and environmental factors such as air resistance. One system that relies heavily on elasticity is the Newton’s Cradle. We aimed to determine the extent to which these adhesives serve to mitigate or worsen the chaotic movements and elastic collisions.

Dynamic viscosity is a quantity that describes the magnitude of a fluid’s internal friction or thickness. Traditionally, scientists measure this quantity by either calculating the terminal velocity of a falling sphere or the time a liquid takes to flow through a capillary tube. However, they have yet to conduct much research on finding this quantity through viscous damped simple harmonic motion. The present study hypothesized that the relationship between the dynamic viscosity and the damping coefficient is positively correlated.

These authors mathematically deduce a model that explains the interesting (and unintuitive) physical phenomenon that occurs when water falls.

Quantum computers can perform computational tasks beyond the capability of classical computers, such as simulating quantum systems in materials science and chemistry. Quantum teleportation is the transfer of quantum information across distances, relying on entangled states generated by quantum computing. We sought to mitigate the error of quantum teleportation which was simulated on IBM cloud quantum computers.

Here, the authors sought to identify a method to optimize the lift generated by an airfoil based solely on its shape. By beginning with a Bernoullian model to predict an optimized wing shape, the authors then tested their model against other possible shapes by constructing them from Styrofoam and testing them in a small wind tunnel. Contrary to their hypothesis, they found their expected optimal airfoil shape did not result in the greatest lift generation. They attributed this to a variety of confounding variables and concluded that their results pointed to a correlation between airfoil shape and lift generation.

The authors explore how similar exoplanets are to Earth and whether they could be inhabited by humans and other living organisms.

With low-temperature transportation being critical for the progress of research and medical services by preserving biological samples and vaccines, the optimization of cold storage materials is more critical now than ever. The exclusive use of dry ice has its limitations. Notably, it proves insufficient for cold storage during long-range transportation necessary for the delivery of specimens to rural areas. In this article, the authors have proposed a new means of cold storage through the combination of dry ice and ethanol. Upon thorough analysis, the authors have determined their new method as considerably better than the use of pure dry ice across many characteristics, including cold storage capacity, longevity of material, and financial and environmental feasibility.