An Analysis of the Density and Patterns of the Solutions of Diophantine Equations of the Third Power

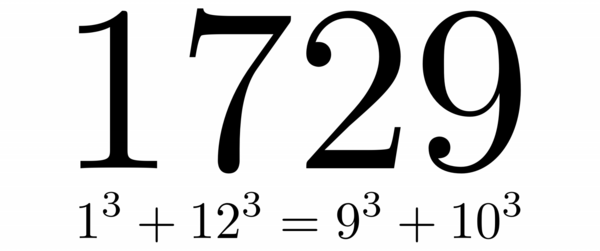
According to Fermat’s last theorem, xⁿ + yⁿ = zⁿ has no solutions if n > 2. We modified Fermat’s equation into the Diophantine equations a³ + b³ + c³ = d³, a³ + b³ + c³ = d² and a³ + b³ + c³ = d⁴ and found their solutions. We analyzed how the density of solutions varied as the numbers got bigger. Ramanujan had devised a formula to find numbers satisfying a³ + b³ + c³ = d³, we compared the density of solutions with those obtained by his formula. We also found perfect cubes, squares or fourth power solutions that could be expressed in different ways as a sum of three cubes. We called them perfect power taxicab numbers. Our hypothesis was that there are many solutions for our equations, and as the inputs become bigger, their density will increase linearly with minor fluctuations. We thought that most perfect power taxicab numbers would have a frequency (number of ways it can be expressed as a sum of three cubes) of two and the maximum frequency would be around 10. We hypothesized that Ramanujan’s formula would give around half of the solutions, and the density of solutions will increase as the numbers become large. We concluded that the density distribution of two equations increases as the numbers become bigger. However, the third equation had a stagnant density. Ramanujan’s formula found many numbers at the start but was unable to reach a high density. One perfect cube taxicab number had a frequency of 42 whereas the majority had a frequency of 2 or 3.